Lesson 19: One-Tail vs Two-Tail Significance Testing

November 7, 2018

Review:

• Significance Testing
• Election Results

Presentation:

• Hypothesis testing: 1-tail vs 2-tail

• 

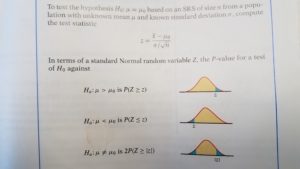
• 2-Tail Tests
  • Example 6.15 (p. 383-384):
    • Ho: μ = 168, Ha: μ ≠ 168, x-bar = 173.7, n=71, σ = 27, α = 0.05
  • Use Your Knowledge 6.43 (p. 385-386):
    • Ho: μ = 25, Ha: μ ≠ 25, x-bar = 27, n=25, σ = 5, α = 0.05
• Compare >, < and ≠
  • Z = -1.73
  • What is the p-value for
    • Ha: μ > μo [use 1 – P(Z)]
    • Ha: μ < μo [use P(Z)]
    • Ha: μ ≠ μo [multiply by 2]

Activity:

Determine whether it’s a 1-sided or 2-sided hypothesis test and solve. Report p-values and determine if you can reject or must fail to reject the null hypothesis.

1. A test of the null hypothesis Ho: μ = μo yields test statistic z = 1.34.
   1. What is the P-value if the alternative is Ha: μ > μo
   2. What is the P-value if the alternative is Ha: μ < μo
   3. What is the P-value if the alternative is Ha: μ ≠ μo
2. The college bookstore tells students the average textbook price is $52 with a standard deviation of $4.50. A group of students thinks the average price is higher. In order to test the bookstore’s claim, the students select a random sample of size 100 and find a sample mean price of $52.80. Perform a hypothesis test to determine if the price difference is significantly higher for α = 0.05.
3. A certain chemical pollutant in the Arkansas River has been constant for several years with mean μ = 34 ppm (parts per million) and standard deviation σ = 8 ppm. A group of factory representatives whose companies discharge liquids into the river is now claiming they have lowered the average with improved filtration devices. A group of environmentalists will test to see if this is true. Assume their sample of size 50 gives a mean of 32.5 ppm. Perform a hypothesis test to determine if the pollution levels are significantly lower for α = 0.05.
4. A manufacturing process produces ball bearings with diameters that have a normal distribution with mean, μ = 0.50 centimeters and known standard deviation, σ = .04 centimeters. Ball bearings with diameters that are too small or too large are problematic.
   1. Assume a random sample n=25 with a sample mean diameter = 0.51 cm. Perform a hypothesis test at α = 0.05.
   2. Assume a random sample n=25 with a sample mean diameter = 0.48 cm. Perform a hypothesis test at α = 0.05.